The standard error of the difference between two means

 As part of the results of an unpaired t test, Prism reports the standard error and confidence interval for the difference between two means.

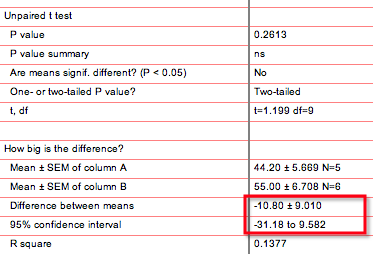
The row labeled 'difference between means' shows just that: The difference between the mean of group A and the mean of group B. It also reports the standard error of that difference. That is used to compute the confidence interval for the difference between the two means, shown just below. 

The standard error for the difference between two means is larger than the standard error of either mean. It quantifies uncertainty. The uncertainty of the difference between two means is greater than the uncertainty in either mean. So the SE of the difference is greater than either SEM, but is less than their sum. With equal sample size, it is computed as the square root of the sum of the squares of the two SEMs. With unequal sample size, the larger sample gets weighted more than the smaller. 

Some people prefer to report SE values than confidence intervals, so Prism reports both. The confidence interval is easier to interpret. Given the assumptions of the analysis (Gaussian distributions, both populations have equal standard deviations, random sampling, ...) you can be 95% sure that the range between -31.18 and 9.582 contains the true difference between the means of the populations the data were sampled from.  

The confidence interval is consistent with the P value. The 95% confidence interval contains zero (the null hypothesis, no difference between means), which is consistent with a P value greater than 0.05. If the 95% confidence interval for the difference between two means does not incclude zero, then the P value will be less than 0.05.